Have you got all of the answers? How important is it to you as a teacher, that when you walk into a classroom you already know the answers to the problems that your students will be working with?
I find this an interesting question – I have heard (and probably provoked) many an animated discussion among teachers who passionately take seemingly opposing views.
Of course, the first issue with this question is context, or lack thereof. The term ‘answer’ is particularly problematic. What does it actually mean? The result? The facts? The end-point of a mathematical question or problem? What about the process of reaching that solution, the strategies employed - in other words - the journey?
And even this is not clear-cut, as it is likely to depend on the reasons why a particular problem or question has been selected for a lesson and what type of problem it is, which in turn depends on the teacher’s intended outcomes of that lesson.
So let’s take an example and compare thoughts. What preparation would you typically do before using this problem with students and why?
What is an equation for the blue parabola?

Based on the variety of responses I have heard in the past, it is interesting to consider the pros and cons of the following connected, but distinct ways of using a rich problem with students. Where does your response in relation to the problem above sit and why? Here is an infographic looking at some of the possible issues:
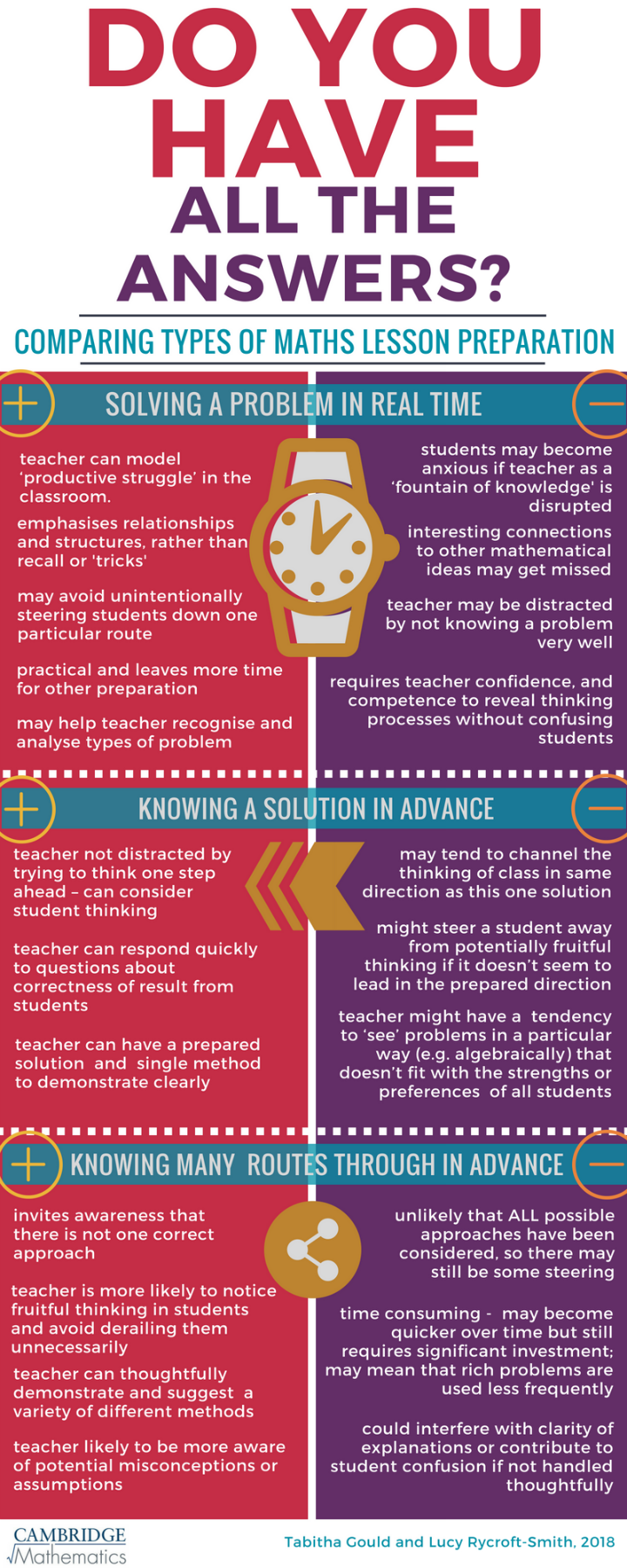
Perhaps a more important question to ask is: “Why do I want students to encounter this problem?”
Is it in order to reach or re-discover a particular result (or relationship)? Is it in order to practise a particular skill (in which case perhaps the choice of a particular strategy will be more important)? Is it to develop mathematical behaviours such as reasoning, resilience, and problem solving? Maybe it is for all of these reasons. Whatever the reasons, the way in which you prepare to use a rich problem will be guided by these bigger ideas.
As a final thought it is worth remembering that, no matter how much preparation you do, you can never predict exactly what each student will do and how they will think when faced with a problem. I chose to use the quadratic problem from above with a visiting group of year 12s. In the process of asking them to feed back some starting points and initial ideas to the group, I was caught completely off-guard by a student who described this:

They proceeded correctly to find an equation for the parabola, but my mind was playing catch-up! I hadn’t expected this and honestly, in the moment, had no idea how to respond.
Needless to say, I went away and thought about it for some time. It is an interesting approach, likely taken because that student had recently begun studying differentiation in their regular mathematics classes. There are some potentially very important ideas that I would want to follow-up if I were teaching that class again. More importantly for me was the reminder that despite thinking that I knew that problem inside-out, I could still be caught off-guard. Being prepared doesn’t, and can’t, mean knowing exactly how that lesson will play out!
The approach now appears as a part of the resource Name that graph again, on the Underground Mathematics website.
