Proportional reasoning

What does research suggest about the development of proportional reasoning in mathematics learning?

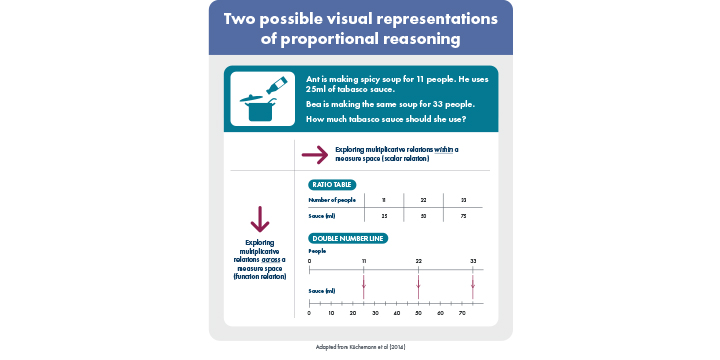
Proportional reasoning involves an understanding of proportionality: change and invariance in relationships, and should be integrated and connected across mathematical strands
Allowing students a wide range of proportional reasoning experiences over a number of years, beginning when they are young alongside development of their rational number sense, is recommended
Proportional reasoning is more than just finding missing values; it is a lens for problem-solving that lays important foundations for algebraic thinking
Premature memorisation of rules is likely to inhibit development of proportional reasoning
Students should have opportunities to sketch, describe and represent proportion problems and relationships between quantities in informal, invented ways before moving towards symbols and algebra
Students should be encouraged to explore different areas of mathematics (including slope, scale, probabilities, vectors and rates) through a lens of proportional reasoning, finding the connections and similarities