Mathematics as an ethical practice

A dice with an arrow pointing forwards, one to reverse and the words stop on each visible face. Placed in a 5 way crossroad

We may think about the art of thinking mathematically as the art of solving problems, and of course “problem-solving” has become part of our vernacular in mathematics education. But mathematics does not have a monopoly on solving problems, and solving mathematical problems is almost certainly not enough (neither necessary nor sufficient) to make us good humans. In fact, sometimes there may be tensions between being a good mathematician and good person – and some have persuasively argued that we are exacerbating this by our mathematical milieus, our house rules, our cultures of doing-mathematics-like-this.

What might those tensions look like?

Imagine a situation where a professional mathematician is asked to:

help design a building so that it is as fire safe as possible
find ways to reduce homelessness in a particular area
refine an algorithm that chooses which court cases to prioritise when scheduling
calculate the upper limit of weight that may be safely borne by a vehicle
set insurance premiums, taking a variety of factors into account

In each of these situations, one could argue that alongside, in between and even within the mathematical problem solving, ethical questions exist. These ethical questions relate to the consequences on human lives that ensue from the mathematical decisions made, and the crucial need to ensure that important information is gathered before, during, and after the problem is solved in order to take these consequences into account. Quite often these questions might need more knowledge than that which is explicitly mathematical – psychology, sociology, biology, geography, economics, engineering, product design – which is why these arguments often sit alongside the urgent call for mathematicians to be more open and collaborative in their work, acknowledging that these contextual questions require input from other experts as well as those affected by decisions. This may be an affront to our conception of mathematics as the seat of pure, rational thoughts; it may feel “messy;” it may seem as if the mathematics is being diluted or sullied by the imperfect contexts in which it is applied. But, as we have seen, the alternative is racist algorithms in hand wash dispensers,¹ mothers being unfairly jailed for killing children they are mourning for,² and skewed systems of measurement that result in seemingly bizarre behaviour as part of “gaming” those systems.³

I attended a recent talk by Prof Paul Ernest⁴ in which they suggested that mathematics was overvalued: “maths runs the world...based on money, reason and rationality, and ideas of utilitarianism.” At first glance, it is easy to take issue with the “overvalued” part – of course we should make decisions based on rationality. What might be the alternatives? But experts in ethics in mathematics offer a coherent argument here: mathematics is only half of the story, and beginning and ending with it can do active harm. Prof Maurice Chiodo puts it thus:

It is easy to create harm as mathematicians. In fact, our very good intentions give us a false sense of security. There are many documented instances of mathematicians doing harm, because defining the problem is the hardest part of the problem – and we often rush that part in our wish to get going.⁵

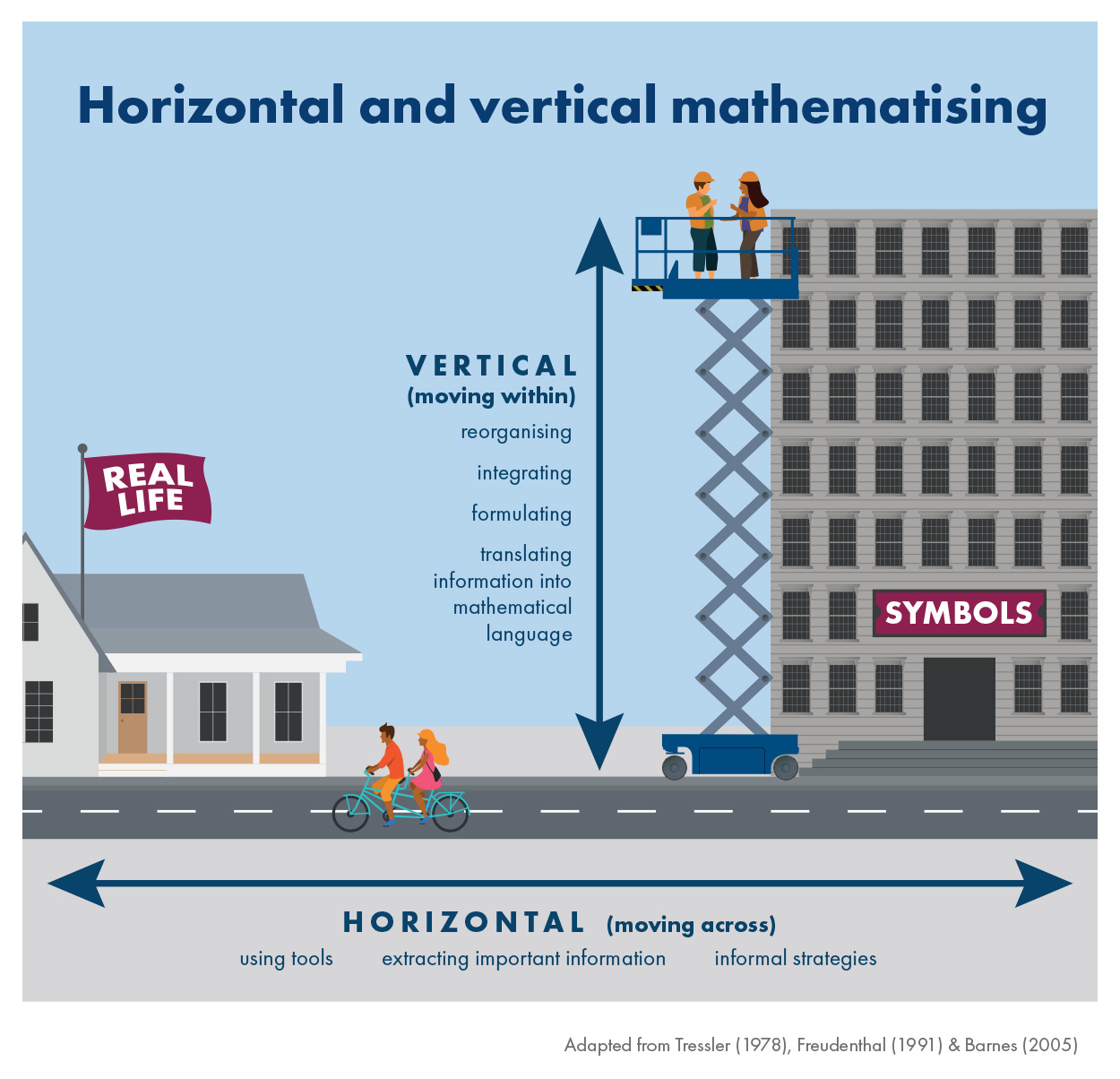
These ideas relate to staying connected with the context, something the RME (Realistic Mathematics Education) movement has been advocating for many years.⁶ The concept of mathematising, shown below, suggests that developing expertise in problem-solving is built on flexibility in moving in and out of different levels of abstraction, language, context and detail. It is not enough to extract and simplify ideas when problem-solving – we have to be able to move in the various other directions too, recontextualising and testing our ideas and strategies as we refine them. In real life, we define problem solving as improving a situation; no matter how good a mathematician we are, if we leave a situation worse than when we started, have we really accomplished anything?

Showing horizontal and vertical mathematising in an infographic

Prof Anne Watson⁷ also suggests an alternative: the principle of ethical care. They suggest that “care is missing, not in individual practice [in maths education], but in the structures in which we have to work.” “Caring is possible in maths education,” Watson says. “It is paying passionate attention.”

Another person who has made suggestions as to a way forward here is Prof Eugenia Cheng. Their book X + Y,⁸ suggesting a move towards a more “congressive” (collaborative/supportive rather than competitive/selfish) model of mathematical practice is convincing, and accords with Chiodo’s “we need to move from asking ‘how can I solve this’ to ‘how can this be solved’.” Cheng says there is “a sharp opposition between those who think as an individual and those who think as a community” in mathematics. Cheng suggests “with the ideas of ingressive and congressive behavior, we can free ourselves...and consider how to make more congressive structures in society, which will stop us favoring counterproductive ingressive behavior.”

Cheng also highlights one of the most important consequences of this change: the type of mathematical and non-mathematical behaviour that gets valued. “If we focus on the congressive aspects of maths, we might notice that the types of people we have previously associated with mathematical brilliance (for example, men who talk themselves up a lot) are being overvalued.”

Prof. Francis Su’s book Mathematics for Human Flourishing⁹ brings these ideas together. Mathematics is about reason, but also about virtue – they offer “a grander, more purposeful vision of mathematics” which explicitly considers the aim of primum non nocere – first, do no harm – and then takes as its focus the principles of exploration, meaning, play, beauty, permanence, truth, struggle, power, justice, freedom, community, and finally (and unapologetically) love. Su suggests that we need to “name a purpose for mathematics that is more than just memorizing procedures for an eventual career...shifting away from contextless portrayals of mathematics to reveal its social and cultural dimensions.” Why learn mathematics – or indeed anything – if we are not using it to make the world better, in ways both large and small?

References:

Join the conversation: You can tweet us @CambridgeMaths or comment below.